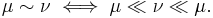Equivalence (measure theory)

In mathematics, and specifically in measure theory, equivalence is a notion of two measures being "the same".

Definition

Let (X, Σ) be a measurable space, and let μ, ν : Σ → R be two signed measures. Then μ is said to be equivalent to ν if and only if each is absolutely continuous with respect to the other. In symbols:

$\mu \sim \nu \iff \mu \ll \nu \ll \mu.$

Equivalence of measures is an equivalence relation on the set of all measures Σ → R.

Examples

Gaussian measure and Lebesgue measure on the real line are equivalent to one another.
Lebesgue measure and Dirac measure on the real line are inequivalent.

References

Halmos, Paul R. (1974). Measure Theory. Springer. p. 126. ISBN 0387900888.